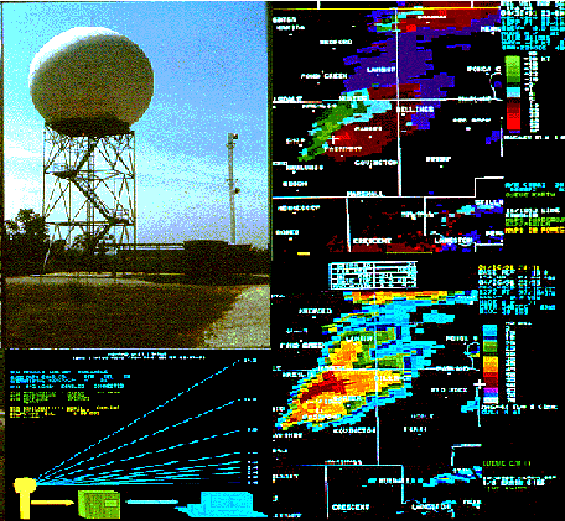
Introduction to Naval Weapons Engineering

Advanced Radar Systems

Radial Velocity Discrimination

In many circumstances, it is beneficial to know both the range and the radial velocity of the target. Since the relative radial velocity is the range rate, a measurement of the radial velocity can be used to predict the target's range in the near future. For example, it allows the prediction of when a target will be inside the effective range of a weapon system. Radial velocity discrimination can also be used to eliminate unnecessary targets from the display. For example, sea clutter or buildings. There are three methods used which can give simultaneous measurement of range and range rate.

Differentiation

This system simply measures the range at fixed intervals and computes the rate of change between the measurements. For example, if a target is at 1500 m for the first measurement and at 1492 m for the next measurement made 1 sec later, the range rate is -8 m/s. Light detection and ranging (LIDAR) systems use this method. Accuracy is improved by taken several quick measurements and computing the average rate of change. The intervals cannot be chosen to be too small however, since the target must be able to change range during the measurement interval.

Moving Target Indicator (MTI)

This system measures changes in the phase of the returned signal to determine motion of the target. In order to measure the phase, a sample of the transmitter pulse is fed into a phase comparator, which also samples the return signal. The output of the phase comparator is used to modulate the display information. Returns will be the largest and positive when they are in-phase the largest negative value when out of phase.

Figure 1. Phase comparison
output.

When the range to a target is changing, the phase comparison output will be varying between its extreme values, as well as moving in range. One full cycle of phase shift is completed as the range changes by one-half wavelength of the radar. This is because the radar signal travels both to and from the target, so that the change total distance traveled by the radar pulse changes by a factor of two. For a typical radar wavelength of 3 cm, it is clear that the phase comparison output will be rapidly varying for targets whose range is changing.

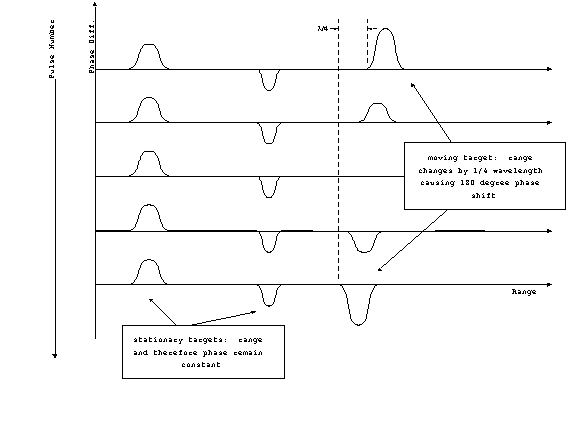
Figure 2. Five sequential
returns from pulse comparison output.

The fact that stationary targets have a fixed value of phase difference can be exploited to remove them from the display. This is accomplished by a cancellation circuit. The MTI processor takes a sample from the phase comparison output and averages it over a few cycles. Moving targets will average to zero, while stationary targets will have non-zero averages. The average signal is then subtracted from the output before it is displayed, thereby canceling out the stationary targets.

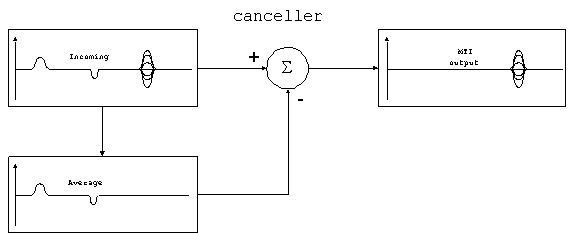
Figure 3. Cancellation
circuit of MTI processor.

What is meant by stationary targets are those returns which are not changing in range. For moving transmitters, of course, returns from fixed objects on the ground will be changing in range and therefore displayed. MTI systems for moving transmitters must provide a modified input to the phase comparator, which includes the phase advance associated with the motion of the transmitter.

Pulse Doppler Radar

This system adds additional processing equipment to the basic pulsed radar system. A sample of the transmitted signal is directed to mixer, which also samples the output from the receiver. The output of the mixer is the Doppler shift, Df. The Doppler shift is passed to a filter which modifies the display information accordingly.

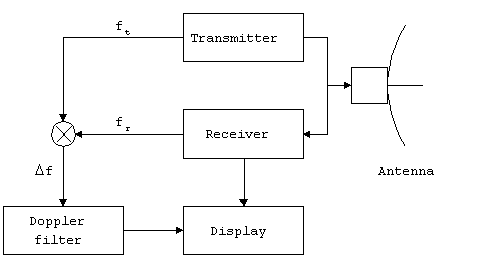
Figure 4. Pulsed Doppler radar
system.

The most common application is to color code the return information on the PPI display. The Doppler shift is sorted into categories, for example positive, zero and negative, which are then associated with colors. In this example, only three colors are used: white, grey and black.


Pulsed Doppler radar systems are used in numerous miltary applications. They are also the standard weather radar throughout the country. The pulsed Doppler radar can detect and graphically display information about the relative motion of winds inside of storm cells and has proved useful in detecting tornadoes. A Doppler velocity display of a tornado will show the two colors which correspond to opposite directions of motion side-by-side.

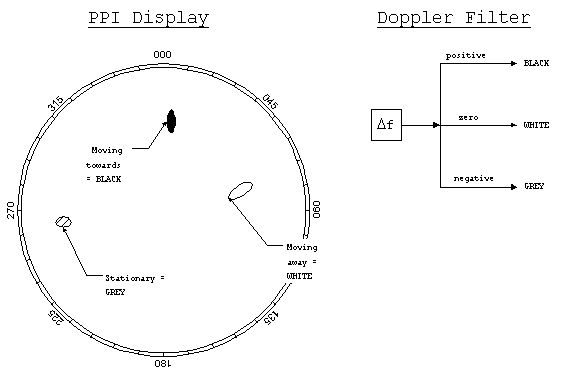
Figure 5. Pulsed Doppler
display.

Limitations

MTI and pulse Doppler radar systems cannot measure velocities above a certain value, known as the first blind speed or maximum unambiguous speed. There are two ways to understand this phenomenon. For MTI systems, the first blind speed occurs when the change in range between pulses is exactly one-half of the wavelength. This changes the phase by 360⁰ which is the same as 0⁰, or no phase shift at all. The target moving at the first blind speed will appear to be stationary and be canceled from the display. Since this condition will only be temporary, it is of no concern.

In pulsed Doppler systems, the radial velocity cannot be measured above the first blind speed. Consider the spectrum of the pulsed Doppler radar system. There will be a main lobe centered on the radar carrier frequency. The bandwidth (BW) of the main lobe will be determined by the pulse width (PW), from the fundamental relationship:

BW = 1/(2PW).

Because the cycle is repeated at a frequency equal to the PRF, there will additional copies of the main lobe at multiple intervals of the PRF on either side of the carrier frequency. In fact, all of the information from the return will be repeated at intervals of PRF, including the Doppler shifted return.

Figure 6. Spectrum of
pulsed Doppler radar.

The only useable portion of this spectrum is the interval between the main lobe and the first harmonic at f_c + PRF. Therefore only Doppler shifts that fall within this range can be measured unambiguously. Therefore the condition when the Doppler shift is equal to the PRF defines the maximum unambiguous speed that can be measured. From this we derive the maximum unambiguous speed:

Df = 2s/l = PRF

S_unamb = lPRF/2

You will note that this is also the same condition described for MTI systems, namely when the target moves one-half wavelength in the period PRT:

s_blind * PRT = l/2 s_blind = lPRF/2.

From the spectrum, it is also apparent that the Doppler shift must be larger than the bandwidth of the main lobe in order to be detectable. This defines a minimum detectable speed:

Df_min = 2s_min/l = 1/(4PW)

s_min = l/(8PW).

Example: WSR-88 (NEXRAD) weather radar.

This system operates at 3 GHz (l = 10 cm) and uses a 325 Hz PRF in its normal mode, find the maximum unambiguous speed this system can measure.
S_unamb = (0.1 m)(325)/2
s_unamb = 16.25 m/s or about 37 mph.

There are two ways to fix this problem. The first is to increase the PRF. Of course, this will reduce the maximum unambiguous detection range accordingly. The other is to vary the PRF. Ambiguous returns will vary either in range or velocity, while accurate ones will not. This does not solve the problem, but can be used to identify conditions where the target range is beyond R_unamb or when the target radial speed is beyond s_unamb.

High Resolution Radar

Pulse Compression

This is a method which combines the high energy of a long pulse width with the high resolution of a short pulse width. The pulse is frequency modulated, which provides a method to further resolve targets which may have overlapping returns. Since each part of the pulse has unique frequency, the two returns can be completely separated. The pulse structure is shown below:

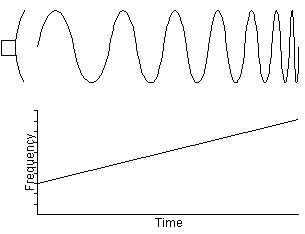
Figure 7. Pulse compression using
frequency modulation.

The receiver is able to separate two or more targets with overlapping returns on the basis of the frequency. Here is a sample return showing two targets with separation less than the conventional range resolution:

Figure 8. Overlapping returns
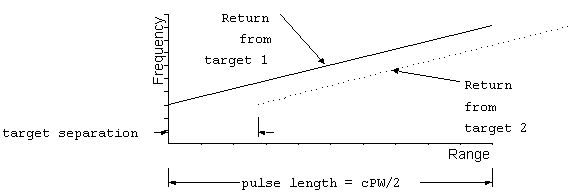
separated by frequency.

When the pulse is frequency modulated in this manner the process or resolving targets on the basis of frequency is called post-detection pulse compression, or PDPC. The ability of the receiver to improve the range resolution over that of the conventional system is called the pulse compression ratio. For example a pulse compression ratio of 20:1 means that the system range resolution is reduced by 1/20 of the conventional system. Alternatively, the factor of improvement is given the symbol PCR, which can be used as a number in the range resolution formula, which now becomes:

R_res = c PW/(2 PCR)

The minimum range is NOT improved by the process. The full pulse width still applies to the transmission, which requires the duplexer to remained aligned to the transmitter throughout the pulse. Therefore R_min is unaffected.

Synthetic Aperture Radar (SAR)

We have already seen that the angular resolution is determined by the beamwidth of the antenna. At a given range, R, the ability to resolve objects in the cross-range direction, known as the cross-range resolution, is calculated by

DR_cross = Rq

where q is the beamwidth expressed in radians. This is merely the arc length swept out by the angle q at radius R. It is also the width of the radar beam at the range R. For example, a 6⁰ beamwidth (0.1 radians) will be 10 m wide at a range of 100 m. For most radar antennas the beamwidth is sufficiently large so that the cross range resolution is fairly large at normal detection ranges. As such, these systems cannot resolve the detail of the objects they detect.

Synthetic aperture radar (SAR) uses the motion of the transmitter/receiver to generate a large effective aperture. In order to accomplish this, the system must store several returns taken while the antenna is moving and then reconstruct them as if they came simultaneously. If the transmitter/receiver moves a total distance S during the period of data collection, during which several return pulses are stored, then the effective aperture upon reconstruction is also S.

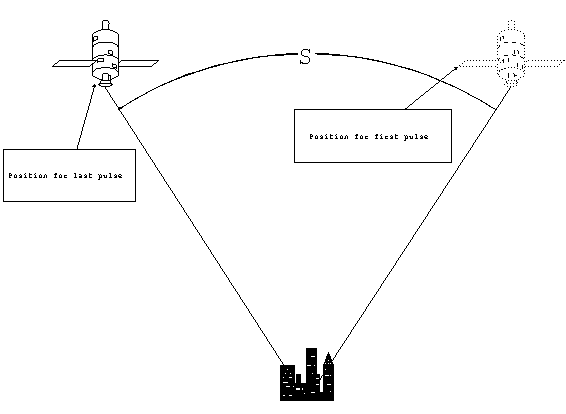
Figure 9. Synthetic aperture.

The large synthetic aperture creates a very narrow beamwidth which can be calculated by the usual beamwidth formula, substituting the synthetic aperture for the physical antenna aperture. The new beamwidth can be used to predict the improved cross-range resolution:

DR_cross R l/S (SAR)

where: R = target range, S = distance traveled by the transmitter/receiver during data collection, and l = the wavelength of the radar.

The most frequent application of SAR is with satellite radar systems. Because the satellite is traveling at high velocity, the accuracy of these systems can be made very high. Furthermore, if the target is fixed in location, the period for data collection can be made very long without introducing significant error. Therefore satellite SAR is used for the imaging of fixed objects like terrain, cities, military bases, etc.

Inverse Synthetic Aperture Radar (ISAR)

It is possible to achieve the same large synthetic aperture without moving the transmitter/receiver. If the target rotates by a small amount, it has the same effect as if the transmitter/receiver were to travel a distance equal to the arc length at the range R. The figure below illustrates this effect for a yaw angle y of a ship at range R.

Figure 10. Equivalence
of SAR and ISAR.

Since the effect is the same we can take over the same results for the cross range resolution, but this time we substitute the distance Ry for the aperture.

DR_cross = R (l/Ry), the range cancels out to give:

DR_cross = l/y (ISAR)

which is a remarkable result, because it is independent of range!

Example:

Find the cross-range resolution of an ISAR system at 3 GHz, that collects data over a yaw angle of 6⁰ (0.1 radian).
The wavelength, l = 10 cm, so the cross-range resolution is
DR_cross = 0.1 m/0.1
DR_cross = 1 m, at any range.

ISAR systems are typically used for long-range imaging and identification of possible targets. The ISAR platform may be fixed or moving. The best targets for ISAR are ships which tend to yaw periodically in the sea state.

Phased Array Radar

It is possible to form a radar beam using a planar array of simple antenna elements (i.e. dipole antennae). It is easiest to visualize this system starting with a linear array.

Figure 11. Linear array of antennae.

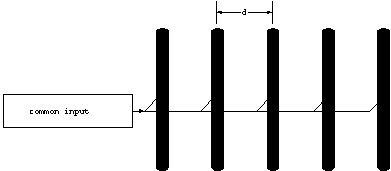
If all of the antennae are driven coherently, meaning at the same frequency and phase, then the condition of maximum constructive interference will occur in the two directions perpendicular to the array axis. Now, if this system is modified to include a variable phase shift on the input to each element, the condition of maximum constructive interference can be changed.

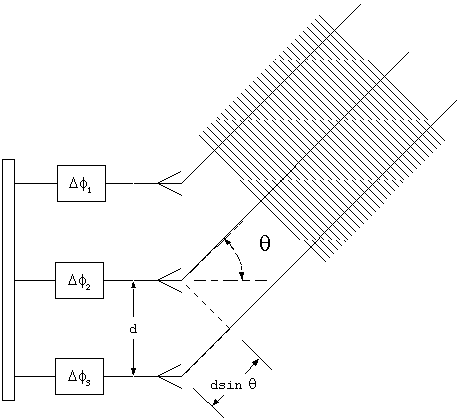
Figure 12. Path length
difference for beam steered off axis.

Here we have a three element linear array, all fed from a common source, with a variable phase shift at each element. In order to change the direction of maximum constructive interference by the angle, q, the phase shifts must be chosen to exactly compensate for the phase shift created by the extra distance traveled, d sin(q). The condition for the phase shift between adjacent elements can be found from

Df_adj = (2p/l) d sin(q)

Care must be taken to assign an appropriate sign to the phase shift. In the example above, the phase of the signal from antenna element 3 must be advanced relative to element 2, therefore the phase shift is positive.

This same principle may be applied to a planar array. In this case, the phase shift will steer the beam both in azimuth and elevation. The phase shifts required may be computed independently and combined algebraically to give the net phase shift required. First we must establish the coordinate system. When facing the array, the upper leftmost element will be the reference with coordinates (0,0). The elements will be assigned coordinates with the first number representing the elevation and the second the azimuthal element number. The general element coordinates will be (e,a), where:

e = elevation element number
a = azimuthal element number.

When referring to the phase shift at a specific element, it will be in reference to the element (0,0). Using this system, we chose the angles to be positive in the directions indicated below:

Figure 13. Planar phased array.

The phase shift required to steer the beam to an elevation angle f (defined so that upwards is positive) and azimuthal angle q (positive when to the left as seen looking into the array), will be

Df_e,a = (2p/l)[ e d_e sinf + a d_a sinq ]

where d_e and d_a refer to the element spacing in the vertical and horizontal directions respectively.